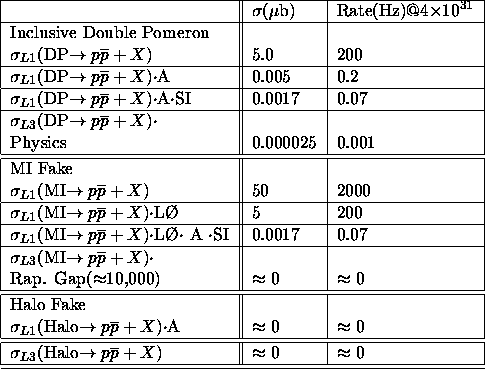
 of exposure
with a 25% efficiency, 25% acceptance (averaged over p's and
of exposure
with a 25% efficiency, 25% acceptance (averaged over p's and
We will also be able to make absolute cross section measurements.
This requires accurate knowledge of our acceptance, and
is where the full power of the FPD is demonstrated.
By using symmetric pots (up-down or left-right), we can
determine the beam position at each quadrupole station
(Sec. 5.1) to an accuracy of about 100 ![]() m.
Averaging the cross sections measured in symmetric spectrometers
reduces the cross section error from the beam position to a
second order correction (which contributes less than 5% to the
total acceptance uncertainty).
We can then use well-measured tracks in the quadrupole spectrometers
to calibrate the dipole spectrometer (for tracks with |t|>0.5 GeV
m.
Averaging the cross sections measured in symmetric spectrometers
reduces the cross section error from the beam position to a
second order correction (which contributes less than 5% to the
total acceptance uncertainty).
We can then use well-measured tracks in the quadrupole spectrometers
to calibrate the dipole spectrometer (for tracks with |t|>0.5 GeV![]() we have a large overlap between anti-protons in the two types
of spectrometers). Conversely, we can use the |t| distribution measured
in the dipole spectrometer (which has full |t| acceptance for
we have a large overlap between anti-protons in the two types
of spectrometers). Conversely, we can use the |t| distribution measured
in the dipole spectrometer (which has full |t| acceptance for
![]() ) to extrapolate the quadrupole measurements to |t|=0.
The dominant error in the acceptance is due to the dispersion of the beam,
which causes about a 15% uncertainty integrating
over the |t| range of the quadrupole spectrometers.
We can reduce this error significantly by measuring the dispersion using
elastics and then unsmearing the |t| distribution. We expect final errors
on the cross section due to acceptance
of a few percent in the dipole spectrometer and less than 10% in the
quadrupole spectrometers.
) to extrapolate the quadrupole measurements to |t|=0.
The dominant error in the acceptance is due to the dispersion of the beam,
which causes about a 15% uncertainty integrating
over the |t| range of the quadrupole spectrometers.
We can reduce this error significantly by measuring the dispersion using
elastics and then unsmearing the |t| distribution. We expect final errors
on the cross section due to acceptance
of a few percent in the dipole spectrometer and less than 10% in the
quadrupole spectrometers.
For a fixed |t| bin, the error in the diffractive mass is ![]() (6% for
(6% for ![]() , 3% for
, 3% for ![]() , etc.). Therefore,
from the FPD point of view we will be able to make accurate
cross section measurements.
For jet
cross sections we will be limited by energy scale errors which will
likely be 30-50% for our
, etc.). Therefore,
from the FPD point of view we will be able to make accurate
cross section measurements.
For jet
cross sections we will be limited by energy scale errors which will
likely be 30-50% for our ![]() range. Using ratios will
allow us to reduce these errors.
This time we will
measure the fraction of jet events above an
range. Using ratios will
allow us to reduce these errors.
This time we will
measure the fraction of jet events above an ![]() threshold that
are diffractive (similar to the rapidity gap measurements), making
us insensitive to energy scale errors. We thus expect to
measure all cross sections (or ratios of cross sections) with errors
of 10% or less.
threshold that
are diffractive (similar to the rapidity gap measurements), making
us insensitive to energy scale errors. We thus expect to
measure all cross sections (or ratios of cross sections) with errors
of 10% or less.